Quantifying Risk and Reward in the Markets

What do we really mean when we say "risk"? Does that have any quantifiable measure?

When Somebody Says That a Certain Stock or Strategy is Risky What Do They Mean?

After all, in the investing world this term gets thrown around a lot. It is (or should be) one of the most fundamental parameters in portfolio construction. And yet, no one really ever talks about what “risk” actually is.

The initial thought that comes to mind is that risk is the term used for the possibility of great financial loss. This would make sense too, because this is the way we define risk in other non-financial related activities. We would say that driving over the speed limit or in bad weather is more risky than driving normally because it elevates the possibility of harm to oneself or others.

However, in investing it doesn’t really equate as well as you would think. Say we have a portfolio that loses 75% of its value and stagnates for ten years before rebounding thousands of percent. Is that considered risky? Because if you held the portfolio to “term” then you would have made out handsomely. Conversely, if we had sold sometime during those ten years then we would have declared the portfolio as “risky” because it incurred a massive loss. How can a portfolio be risky as well as not risky just simply based off of the time frame of the investment?

This is why the concept of risk in the traditional sense, is flawed when it comes to investing.

That’s also why when Modern Portfolio Theory came about, they cleared that aspect up. They defined risk as “uncertainty” or “deviation” from the mean. And as we may now well now, the “mean” is just the expected return of the portfolio.

So basically, the greater the deviation or swings in the value of our portfolio the greater the risk. That’s at least what modern portfolio theory posits.

So How Do We Measure Risk?

Well, since risk is just defined as the deviation from the mean we can use standard deviation to measure our risk. If you’re not familiar with standard deviation you can check out our last article where we discuss it in depth.

But basically in a nutshell, standard deviation just gives us range in which an asset or portfolio is likely to fluctuate within. The lower the standard deviation, the less intense the fluctuations.

So a portfolio that averages 10% a year with a standard deviation of 8% will have returns between 2% and 18% in any given year 68% of the time (68% specifically because that is the probability of the first standard deviation).

Great! So now that we have that cleared up why don’t we try an experiment?

The Efficient Frontier

What if we tried plotting our returns against our risk?

You might say, why bother? We know that more risk will generally mean more return right?

And you would be correct, but the question is, is the relationship between risk and return linear or curved? Because if the relationship is linear then yes, there is no further questioning needed. The risk reward profile would look like this.

X axis is risk Y axis is return

However, if the relationship isn’t linear, then we can optimize a portfolio to get the most return for the least amount of risk.

For example, if the relationship looked like this…

X axis is risk Y axis is return

Then everybody would be taking as much risk as possible because the returns would magnify exponentially! So obviously, this curve wouldn’t mirror reality.

So how can we figure out what (or whether there even is) the curve for the risk reward tradeoff? Well, we could model a bunch of random portfolios and then measure their standard deviation and returns to plot on a graph, which luckily someone has done exactly that.

This is the curve we get when plotting risk against reward, and if you pay careful attention, you’ll realize that only the edge of the curve has the most optimized returns. Anything inside the curve has a less than ideal risk to reward ratio. You also may notice that the curve favors the risk side when we get above about a 10% return. For every extra percentage point of return we receive we are taking on an even larger portion of risk. This is unlike the earlier part of the graph (from a 5-10% expected return) which shows that we get more return than risk. If you want to read more about this you can check out this medium article where they use Python to calculate the efficient frontier.

The Importance Of The Sharpe Ratio

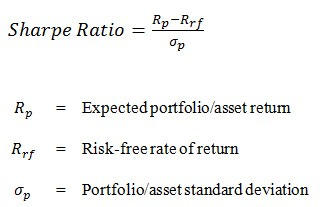
Now as we mentioned before, since the efficient frontier isn’t linear it means that there is an optimal point on it where we get the most return for the least risk. We can find this using something called the Sharpe ratio. The formula for calculating it looks like this.

The higher the Sharpe ratio is, the more optimized the returns are for the risk.

Some Parting Thoughts…

The efficient frontier will always have the “sideways parabola” look to it. However, the exact curvature of it can shift based on the assets and strategies we are using. Our hope is that we could find a strategy that lies outside the efficient frontier for a passive indexing approach to investing, because it would mean that no combination of assets could match its Sharpe ratio.

Another interesting thing to note, if you think about it, a Ponzi scheme also lies outside of the efficient frontier because they have low volatility (until it comes apart obviously) and a “good” return.

So basically, if a portfolio/strategy lies outside of the efficient frontier its one of two things

1. An unbeatable strategy

2. A ponzi scheme

I might consider writing an article on using the efficient frontier to spot a Ponzi scheme in the future, but for now, this is all I have.

By the way, if you enjoyed this post, why not share it with a friend? We promise it won’t take long.

Until Next Time!

Disclaimer:

All content is for discussion, entertainment, and illustrative purposes only and should not be construed as professional financial advice, solicitation, or recommendation to buy or sell any securities, notwithstanding anything stated.

There are risks associated with investing in securities. Loss of principal is possible. Some high-risk investments may use leverage, which could accentuate losses. Foreign investing involves special risks, including a greater volatility and political, economic and currency risks and differences in accounting methods. Past performance is not a predictor of future investment performance.

Should you need such advice, consult a licensed financial advisor, legal advisor, or tax advisor.

All views expressed are personal opinion and are subject to change without responsibility to update views. No guarantee is given regarding the accuracy of information on this post